Bereichsnavigation
2. Descriptions of various theoretical schemes of well-tempered tuning
Here I will describe and comment upon some characteristics of several (albeit less than a dozen) quantitative schemes of "good temperament." This phrase is a literal translation of a late-17th- and 18th-century German term, gute Temperatur. Some but not all of the relevant theorists called Equal Temperament "good" (or even "pure," rein); and, some of those who did so – including Andreas Werckmeister in his later years – also said or implied that certain forms of unequal temperament were even better than Equal Temperament. Among the quantitatively formulated schemes for "good" unequal temperament, I have found a general tendency for the inequalities to be as follows in regard to the semitones and major 3rds:
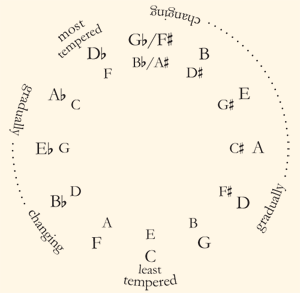
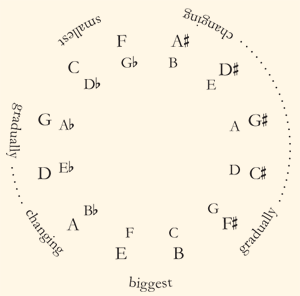
The following descriptions of relevant theoretical schemes will include two kinds of diagrams:
(1) Circular diagrams indicating the sizes of the twelve semitones in terms of a modern unit of measure called a "cent" (defined as 1/1200 of a pure octave, i.e. of an octave produced by two sound-waves whose lengths and frequency ratios are exactly in the ratio 2:1). Most of these diagrams will include numbers showing the difference in size between semitones that are adjacent in the diagram.
(2) Non-circular diagrams indicating how much the various 5ths and major 3rds are tempered. In each of these diagrams the unit of measure is the schisma (as explained in the preceding Webpage), and the four note-names in the top row C♯, G♯, D♯, A♯ - represent the same pitch-classes as the first four in the bottom row (D♭, A♭, E♭, B♭), and the last note-name in each row of five names is for the same pitch-class as the same name at the beginning of the next higher row (F, A, C♯).
(This latter kind of diagram is a flat representation of an involuted diagram which one can imagine being formed by (a) pasting the top row over the first four names in the bottom row to make a tube; (b) joining the ends of the tube to make a toroid but with the tube twisted 120 degrees (before joining the ends) so that the names in the last column of the flat diagram are at the same places as the identical three names in its first column (and the "C♯"s in the flat diagram will be at the same location on the toroid as the "D♭"); and (c) supposing that the distances between one location (representing a pitch-class) and any other neighboring one in the resulting matrix are all the same. I devised this type of involuted diagram in the 1960s. Donald E. Hall and I described it in 1974 in a report, entitled the The musical doughnut, to a national convention of the American Association of Physics Teachers. A sketch of a three-dimensional version of the supposed topography is on page 66 of Ronald Turner-Smith's and my Mathematical Models of Musical Scales.)
The reason for considering so many schemes is that the documentation from Bach's day does not indicate that any one of them (nor indeed any other, similar one) was specifically the one used by Bach, but instead merely shows that a style of tempering advocated by Johann Georg Neidhardt was regarded, by knowledgeable people close to Bach, as better than the scheme for which Werckmeister was already in those days well known. A distinctive feature of my acoustical and musical findings is that the unit of measure for tempering used by Neidhardt in his theoretical formulations is not quite small enough (if, like Neidhardt, one is unwilling to deal in terms of fractions of that unit) to enable one to specify the most suitable nuances (suitable, that is, to the preludes and fugues in Part I of The well-tempered clavier) that a first-rate tuner could readily produce on a harpsichord or not-very-large organ. I therefore conclude that none of the 18th-century German theoretical schemes is quite as finely nuanced as Bach's actual tuning is likely to have been. My reasons in support of this conclusion are described detail below and are illustrated in further detail in the audio-recordings.[1]
Here is a brief account of equal temperament to serve as a point of reference for comparison with the other ones described presently:
Equal Temperament

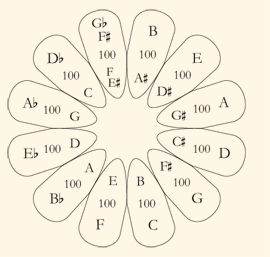
The upper diagram shows that in equal temperament the major 3rds are tempered seven times as much as the 5ths & 4ths. The 4ths and major 3rds are tempered larger than pure, the 5ths smaller. The total amount of tempering for each chain of four 5ths and the resulting major 3rd has to be 11 schismas (in this case, 4 x 1 + 7).
Each major 6th is tempered larger than pure by the sum of the amounts of tempering of the 4th and major 3rd which it comprises, and thus each minor 3rd smaller than pure by the sum of the temperings of the 5th and major 3rd which make a triad with it. I will, for the sake of simplicity, not include here numbers for the amounts of tempering of the major 6ths and minor 3rds, even though they are musically just as important as the major 3rds.
Let me mention here some information of use to readers of my essay,Innovations in temperament and harmony in French harpsichord music, published in the journal Early Music (2013/41: 403-420): Most 16th- and early 17th-century keyboard musicians felt that all the major 3rds should be tempered much less than in equal temperament or should even be pure. The term “1/4-comma meantone temperament” refers to a kind of tuning in which (a) each 5th and 4th is tempered by 1/4 of the syntonic comma and (b) each major 3rd is therefore pure:
In such a tuning on an instrument with no more than twelve notes in the octave, each of the five non-naturals is serviceable either as a sharp or else as a flat, but not both. There would normally be, for instance, a C♯ but no D♭:
![]()
There would normally be a B♭ but no A♯:
![]()
And so on. In “1/5-comma meantone temperament”, each 5th and 4th is tempered by 1/5 of the syntonic comma, and since 11 / 5 = 2.2 the gist of this kind of meantone temperament is:

—which entails:

In any meantone temperament, the diatonic semitones (i.e. the semitones between notes whose names are written with two different letters, for instance F♯-G and A-B♭) are uniformly larger than the chromatic semitones (i.e. between notes whose names are written with the same letter, for instance F-F♯ and E♭-E). Meantone temperament thus provides no variety of melodic nuances among different major and minor keys.
(Technical information about some other kinds of 17th- and 18th-century French-style tuning is provided in the Early Music article.)
Nuanced semitones are aesthetically important for Das wohl temperirte Clavier. These nuances can be inferred approximately by recalling that every diatonic semitone is equal to the difference between a 4th and a major 3rd, so, the more any given major 3rd is tempered, the smaller will be the semitones just above and below it. For instance, if D♭-F is tempered considerably more than is C-E, then C-D♭ and F-G♭ will be considerably smaller than B-C and E-F.
Several 20th- and 21st-century writers on tempered tuning whose research has not included playing the music in different temperaments have argued that Bach is most likely to have performed in equal temperament.
A version of Werckmeister's main scheme of unequal temperament (1680):

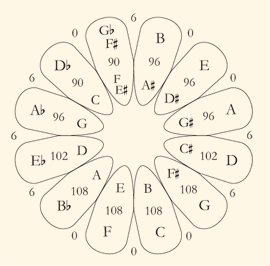
Werckmeister devised this scheme in terms of "1/4-comma" without distinguishing between the Pythagorean comma (≅12 schismas) and the syntonic comma (≅11 schismas). For numerical simplicity I have interpreted his "1/4-comma" in terms of the Pythagorean comma when applied to 5ths. He made two other statements which contradict this inference, however: (1) He said that in this same scheme the least tempered major 3rd, C-E, would be tempered by 1/4-comma, which is in fact distinctly more than the 2 schismas inferred in my diagram; it would have to be 2 3/4 or 3 schismas (i.e. 11/4 or 12/4). (2) He said that organs could be converted to his scheme from the old-fashioned tuning (which in his theoretical reckonings he took to be a meantone temperament with pure major 3rds, whereby all the 5ths would have been tempered by 1/4-syntonic-comma, i.e. by 2 3/4 schismas) by resetting only some of the notes. Obviously he meant that C-G-D-A could be left as they already were. (The other pitch-classes could then be set by tuning pure all the remaining 5ths/4ths except E-B, which might automatically be tempered by about the same amount as C-G-D-A if one took care not to tune any of the pure 5ths larger than pure, because in that case a schisma's worth of tempering might be distributed imperceptibly among the eight ostensibly pure 5ths, and thus the Pythagorean comma would be distributed as follows: 4 x 2 3/4 + 1 = 12 schismas.)
(For documentation of my statements about 18th-century writings on tempered tuning, please see my Stimmung und Temperatur.)
Apart from these rough spots in his account of the scheme, Werckmeister mentioned one of its salient musical defects: that it puts C♯ too high in relation to A and E. Another salient defect is that the tempering of the 5ths is unduly obtrusive, for a combination of two reasons: (1) "1/4-comma" is distinctly more than needed to obtain the desired amount of tempering for C-E; if for instance one interprets that latter "1/4-comma" as 3 schismas, it can be obtained by tempering C-G-D-A-E by only 2 schismas each, since 4 x 2 + 3 = 11; and (2) the rather hefty (by 18th-century standards) tempering of C-G-D-A and E-B in this scheme is rendered all the more salient by the fact that the other two 5ths among the naturals, F-A and A-E, are pure. I imagine that it was these musical aspects of the scheme which caused people close to J. S. Bach to suggest that Neidhardt was better than Werckmeister as a theorist of tempered tuning (but I believe that Bach would consider more nearly satisfactory an organ tuned according to this scheme than one tuned in a meantone temperament).
Dr. Rudolf Rasch argues that Werckmeister in his last years (and therefore Bach) preferred Equal Temperament, but this argument is based on suppressing the fact that Werckmeister's strongest praise for Equal Temperament, which is to be found in Werckmeister's last book (published posthumously in 1707), is followed by an explicit statement that he still preferred a nuanced tuning. The statement is cited in my Stimmung und Temperatur (see above); here is a reproduction of the relevant pages from his book:


Vallotti's scheme (1770s):

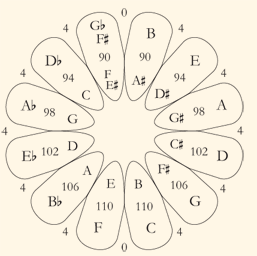
This is very simple (the six 5ths among the naturals are tempered equally, the six others pure) and is even easier than Werckmeister's scheme for an inexpert tuner to set. I therefore included instructions for it in an articlepublished in 1977; but for many years now it has seemed clear to me that in this scheme G♯ and D♯ are each too high in relation to the major 3rds and 6ths below them (and so I presume that Bach and his colleague, Zacharias Hildebrandt, who often tuned for him, would avoid such a defect).
(A substantial account in Italian of Vallotti as a theorist-composer is available here.)
Here is a recording of a Bach chorale performed automatically in this theoretical scheme of Vallotti’s:

A scheme given moderate praise by Thomas Young (1800):

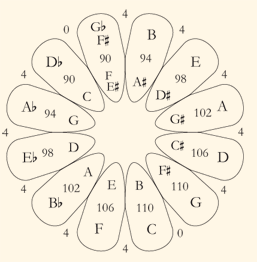
This is conceptually almost as simple as Vallotti's scheme, and it doesn't have the defects of that scheme, but I find it musically defective (I think Bach might have felt likewise) in several ways: F-A is tempered much more than G-B; D-F♯ is tempered no more than G-B; A♭ is a little too low in relation to C, and D♭/C♯ is a little too low in relation both to F and to A.
An approximation to Young's preferred scheme:

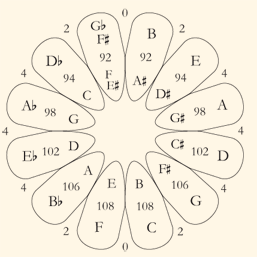
Young suggested that (a) C-E be tempered by 1/4-syntonic-comma (i.e. by 2 3/4 schismas), (b) C-G-D-A-E be tempered uniformly, (c) F-C and B-F♯ be tempered alike, and (d) the other 5ths be tuned pure. The resulting difference in tempering between G-B and F-A is less than in his other scheme, but F♯-A♯ and D♭-F are, in my opinion, still tempered a little too much for Bach's music (and Marpurg would have agreed if he had known about this scheme; he regarded 10 schismas as the permissible limit for even the most heavily tempered major 3rd), and so also is B-D♯.
(Young's prescription for these tunings is on pp. 144-145 of the text to which you can link here)
A scheme recommended by Neidhardt (1724, 1732):


(A notable feature of Neidhardt's method of discussion is that after describing a considerable number of drawing-board schemes he would recommend only a few of them as having practical value, each one for a different kind of venue. A facsimile of the second edition (1734) of his treatise of 1732 is available on the Web, and you can see there that after the drawing-board schemes are described on pp. 26-39, the choice of three musically useful unequal temperaments is made in the lower half of p. 40, and then equal temperament is dealt with on p. 41.)
As in the schemes represented by the previous three pairs of diagrams, so here too the 5ths C-G-D-A-E are tempered uniformly, which is good because the average amount of tempering among them has to be the greatest for any chain of four 5ths in the circle (since C-E is the least tempered major 3rd) and therefore none of them ought to be tempered by more than that average. In a musically good unequal temperament of the kind which all these theoretical schemes were intended to represent, the 5ths should be tempered as unobtrusively as possible. They should sit like polite little girls with their feet crossed and their hands folded in their laps, while the nuanced 3rds and 6ths and semitones make appropriate balletic gestures in the music.
Nevertheless, I judge B♭ to be, in this scheme, too low in relation to D; D♭/C♯ too high in relation to F and to A; and G♯ too high in relation to E. One way to address this latter problem is to tune E a little higher in relation to C as in the scheme represented by the next diagram.
Another scheme recommended by Neidhardt:


It seems to me that while 4 schismas' worth of tempering is not too much for C-E in Bach's music, an expert tuner such as Bach or Hildebrandt would undoubtedly obtain the desired amount for C-E by tempering C-G-D-A-E uniformly rather than by tempering three of those four 5ths twice as much as the other one (as recommended in this scheme). But Neidhardt would never reckon in fractions of his theoretical unit of measurement. So, I regard him as having tried to codify subtleties by means of inadequately subtle theoretical equipment. I think that another indication of this fact in this particular scheme is the lack of nuance among A-C♯, E-G♯ and B-D♯ - not to mention that G-B is tempered much more than F-A, and that D-F♯ and A-C♯ are tempered too much.
An approximation to the semitones and the tempering of the major 3rds in the scheme of Mercadier de Belesta (published in 1793):

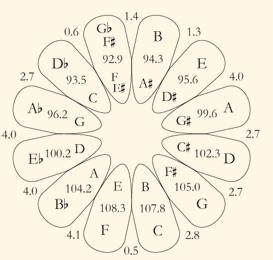
(To include here numbers for the temperings of the 5ths would clutter the diagram with unduly long numbers. Please see p. 255 in my Stimmung und Temperatur, cited above.)
Mercadier's scheme was intended to represent a compromise between equal temperament and the French accord ordinaire. According to Mercadier, C-G-D-A-E were to be tempered uniformly, E-B-F♯-C♯-G♯ uniformly less, and F-C almost the same as this latter chain; hence the pattern of asterisks in my diagram.
The asymmetrical aspect of this pattern in relation to C-G-D-A-E - i.e. the fact that the 5ths in the chain involving the first three sharps (B-F♯-C♯-G♯) are tempered whereas those in the chain involving the first three flats (A♭-E♭-B♭-F) are pure reflects nicely a basic aspect of the 18th-century French style of tuning, and, to a lesser degree, of the contemporary German style as well: namely, that B♭ (let us call it the "first flat") was tempered more in relation to D than was F♯ (the "first sharp"), E♭-G (involving the "second flat") more than A-C♯ (involving the "second sharp"), and A♭-C more than E-G♯. The nuances among the flats were thus more pronounced than among the sharps. A very adequate amount of documentary evidence from 18th-century writings supports this conclusion.
A scheme for which great claims were made in 2005:

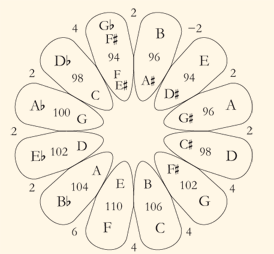
(In the circular diagram, the 'minus 2' between A♯-B and D♯-E means that D♯-E is 2 cents smaller than A♯-B. In the other diagram, the 'minus one' between B♭ and F means that B♭ is to be tempered lower than pure in relation to F. This was characteristic of French but not of German 18th-century tuning. (The French would characteristically temper E♭-B♭ in the same way, and thus E♭-G would be tempered distinctly more than A-C♯.) No conceivable musical purpose is served by tempering B♭ low in relation to both E♭ and F in this scheme. If it were tuned pure to both of those notes, then (a) the amounts of tempering for the following succession of major 3rds: F-A, B♭-D, E♭-G would increase more smoothly, i.e. increasing from 3 to 5 to 7 units instead of from 3 to 6 to 7, and (b) F♯-A♯ would no longer be tempered less than B-D♯.)
This scheme was devised by an American harpsichordist, Bradley Lehman, who undertook to prove in an ambitious article ("Bach's extraordinary temperament: our Rosetta Stone") in Vol. 33 (2005) of the journal, Early Music, that it was invented by Bach and that he had chosen to convey it to posterity by means of a pattern of loops on the title-page of the manuscript of Part I of The well-tempered clavier. The editor of the journal, after declaring in Vol. 33 that the article "clearly establishes the temperament Bach had in mind," published in Vol. 34 a well-received rebuttal ("Bach-style keyboard tuning"). To rehash here the tedious details in regard to the alleged documentary evidence would be gratuitous. Musically it is noteworthy that in this scheme G-B is tempered much more than F-A and that G♯ is tuned too high in relation to E and B (even more than in Vallotti's scheme). You may notice this latter point in Bars 11-13 of Dr. Lehman's recorded performance, available at YouTube, of the C-major fugue from Part I of The well-tempered clavier – and also in the following recordings of a Bach chorale performed – first automatically and then manually – in Dr. Lehman’s temperament:
To track (Lehman's temperament, automatically)
To track (Lehman's temperament, manually)

The unequal temperament used here on the organ:


I designed this scheme in 1994 for a set of recordings to be used in a half-hour presentation. To ensure that the nuances would be effective at first hearing, I made the "stretch" between the extremes between the amounts of tempering for C-E and of D♭-F - slightly greater than if tuning for perennial use. The organ is a small two-manual instrument designed by Charles Fisk in the 1950s. The performer is Peter Sykes, the 1993 laureate of the Erwin Bodky Award for excellence in early-music performance. In recent years this set of recordings has been of use in demonstrating that it is good to make the nuances among the flats more pronounced (as in fact they most often were in the 18th century) than among the sharps.
Here are the precepts that determined the design of this scheme: (1) C-G-D-A-E to be tempered uniformly (and in a way that could be written exactly in terms of the traditional unit of measure without resorting to two decimal places; otherwise the lecture would be clogged by long numbers) to yield more than 3 but significantly less than 4 units of tempering for C-E. (To temper those 5ths by 1.8 units each would have left C-E tempered by 3.8 units, which for my purpose on this occasion would have been too much.) (2) D♭-A♭-E♭-B♭-F to be tuned so as to (a) accentuate the extent to which the nuances among the flats would be more pronounced than among the sharps, and (b) yield a bit more than 10 units of tempering for D♭-F, but (c) without tuning any 5th larger than pure (since that was only a French, not a German device); (3) F-C and E-B to be tempered about half as much as C-G-D-A-E so as to yield a slightly larger amount of tempering for F-A and G-B than for C-E; and (4) the remaining two 5ths tempered virtually alike and thus each about half as much as F-C and E-B (since the sum of tempering in the circle of 5ths has to be 12 units). I let F♯-C♯ be tempered (theoretically) by more than B-F♯ so that F♯-A♯ would be tempered hardly more than A♭-C. Since 1/10 schisma (≅1/5 cent) is, however, at about the lower limit of the amounts by which it is possible to control tuning, it would be more accurate to say, in practical terms, that I took care to ensure that F♯-C♯ not be tempered less than B-F♯, so that F♯-A♯ would not be tempered perceptibly more than A♭-C.
The unequal temperamentused here on the piano:

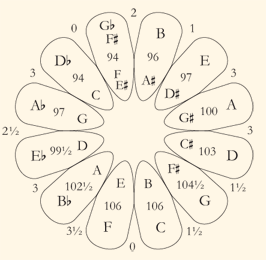
I devised this scheme in 2008 in order to enable an impeccably competent piano tuner, Mitch Staples (in Columbus, Ohio), to produce on a modern concert-grand piano, without any personal instruction from me, a tuning not outside the limits of my concept of Bach-style unequal temperament. (I don't think Bach had this or any other mathematically formulated scheme in mind. I think he tuned by ear.)
In the following diagram all the numbers are twice as big and thus approximate to cents:

The numbers in the top and bottom rows of the following diagram represent my suggestion as to how many cents higher or lower each note should be than its counterpart in Equal Temperament (given a piano already tuned in Equal Temperament, and/or a tuning-machine designed with Equal Temperament as a basis of reference):
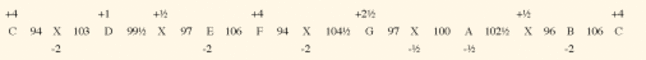
To avoid raising C and F by quite as much as 4 cents, one could make everything a half-cent lower. This would leave E♭/D♯ and B♭ at the same pitch as in Equal Temperament.
Here are some acoustical guidelines for tuning this scheme of unequal temperament with concert-A at about 440 HZ: The major 3rd & 10th between E and tenor's low C should beat exactly 3 times per second. The major 3rd in the bearing-octave between E and G♯ should beat exactly 7 times per second (like F-A in Equal Temperament[2]).The 5th (& 4th) between middle D and A should beat nearly but not quite 2 times per second. The following 5ths (& 4ths) should be tempered as in Equal Temperament: F-C, E-B-F♯-C♯ and G♯-D♯. B♭ should be pure with F. The two 5ths C♯-G♯ and E♭-B♭ may perhaps draw pure, but must nevertheless be on the "shy" side, i.e., definitely not larger than pure. Here are recordings of the Bach chorale in this kind of tuning and (for further comparison) in Equal Temperament:
[1] If you are using a Mac computer and if "Safari" (the Mac browser) doesn’t enable you to play the tracks, please use another browser. On certain kinds of non-Mac computers, this or that browser may cause such a problem or else you may perhaps have to click (on the little triangular white-on-red "play"-sign) with the right part of the mouse and then, in a little window which pops up, click on "Play/Pause".
[2] Professional piano tuners know that F-A in the "bearing octave" (i.e. the major 3rd, F-A, which both tenors and basses can sing) should beat 7 times per second in Equal Temperament when concert-A is at 440 HZ. Here is a slightly rough but perfectly adequate explanation: If concert-A is at 440 HZ (i.e. due to a sound-wave frequency of 440 per second), then the A in the bearing octave is at 220. The beating is caused by mutual interference between sound-waves of the fourth partial tone (at 880) of that A and the fifth partial tone of the F a major 3rd below, i.e. due to an impure unison between those two overtones. Now, the frequency-ratio for a pure 5th is 3:2; for a pure 4th, 4:3; for a pure major 3rd, 5:4; and hence for the syntonic comma (a micro-interval, i.e. too small to serve as a melodic interval), (3:2) x (3:2) x (3:4) x (3:4) / 5:4 = 3^4 : (5 x 2^4) = 81:80. So if our F-A were impure by that latter amount, the beating would be due to the higher of those two overtones (the fourth partial tone of A and the fifth partial tone of F) having a sound-wave frequency per second 1/80 more than the lower one. But 1/80 of 880 is 11, which just happens to be the number of schismas in the syntonic comma; and thus the number of beats per second in an impure consonant harmonic interval when the impurity is due to mutual interference in a unison between overtones at ca. 880 HZ is the same as the number of schismas (which in the case of F-A in Equal Temperament is 7). When the beating is due to an impure unison between overtones at some other pitch level, the number of beats is accordingly faster or slower (e.g. 25% faster if that unison is at the level of soprano's high C♯, 33% faster if it is at the D a semitone above that, 50% faster if it is at the E a whole-tone above that, 50% slower if it is at the level of alto's high A, and so on.) I use this rule to calculate the desired rates of beating of all tempered consonant intervals. It is accurate enough.

